Zoom International Math League: Estimation Challenge Part 2 (Weekly Brain Potion Discussion)

Discussion Forum

Estimation Challenge Part 2 (Weekly Brain Potion Discussion)

Those of you who missed last week's estimation challenge can read about it by clicking here.

For part 2, after drinking all the water in the bottle, John decided to fill it up with the bottle with little spheres, as shown in the picture below:

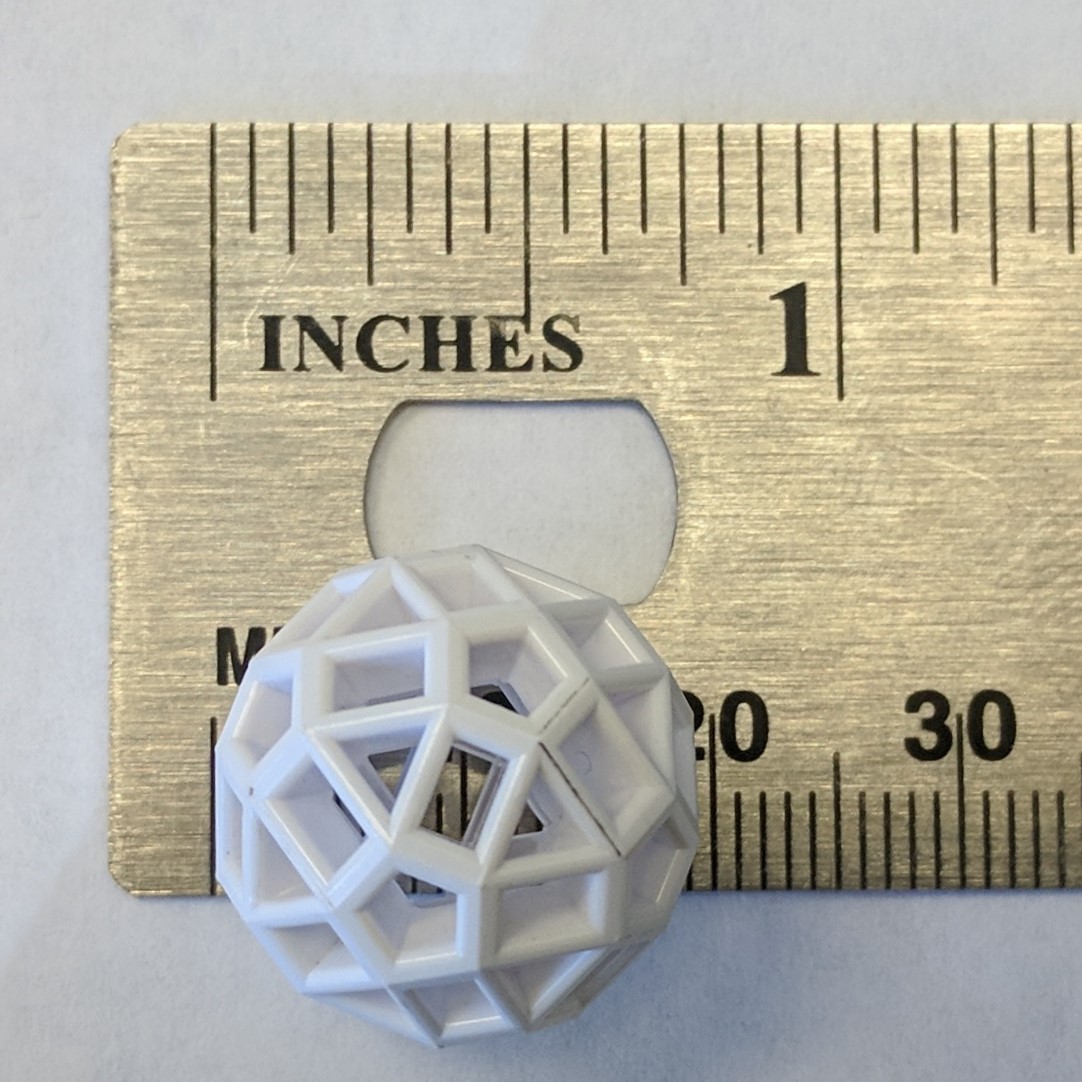
Those of you with good eyes will notice that the objects were not actually small spheres, but actually polyhedra. For practical purposes, they are each roughly a sphere with a diameter of approximately $20$ millimeters:

From part 1 we say the volume of the bottle is approximately $1400$ mL. How many little spheres do you think fit inside the bottle?

Share your answer and reasoning below and we'll announce the person who got closest to the actual number next Friday. Good luck!

40?

顯示上層文章

200

for each of the little spheres, they have an area of 4/3picm^3, which is slightly more than 4cm^3. However, filling up space with spheres wastes a ton of space. A 4cm by 4cm by 4cm box can hold 8 of these, more than 32cm^3 in 64cm^2. Given this, I will say that each of these takes up an average of 7, as those near the edges take up more space, but the ones in the middle are more compact.7 cm^3 is 7 ml, giving us 1400/7, which is 200.

顯示上層文章

Thanks everyone for the participation (and sorry for the delay in the answer).

There were actually $75 + 75 + 75 + 20 + 1 = 246$ of the little spheres inside the bottle. You can see them all lined up in the picture below:

Chris's idea of using the volume of the bottle as well as the volume a single sphere takes up is a good approach!

This problem is one example of what is called a "packing problem". These types of problems, even in two-dimensions can get fairly complicated fairly quickly! Perhaps we'll see another discussion about a "packing problem" in the future!

顯示上層文章