Congratulations to all of the winners for the February 2017 ZIML Competition this past weekend!
I would like to begin and apologize for the handful of typos we have seen throughout the competition. Due to this, it causes a significant amount of misunderstanding in solving some of the problems during the competition. We have rewarded points for those that unfairly received no credit for the correct answers submitted at the time of the exam. If you have any questions or concerns, please let us know.
In this post, we will mention the overall changes that we have made on the February 2017 ZIML Competition.
Division E Problem 4: The 'L' shape below is made up of four $1\times 1$ squares.
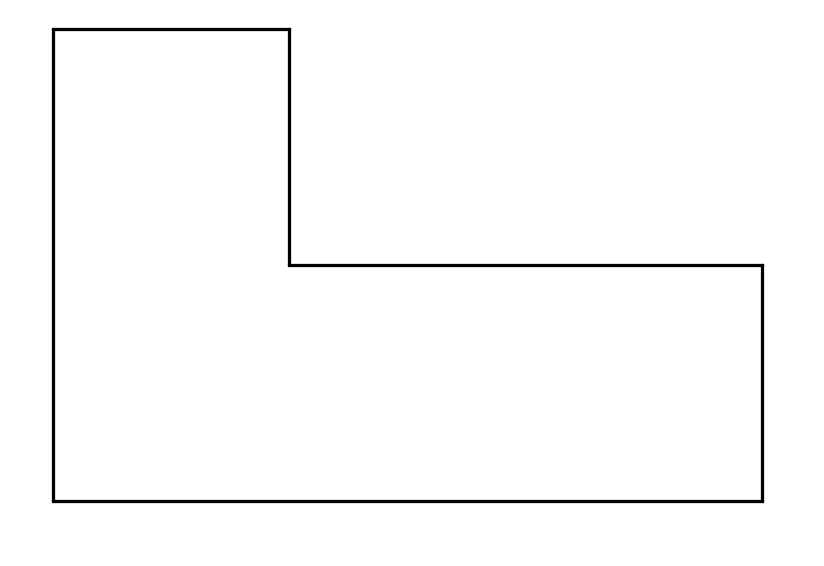
How many shapes of like the one above would you need to cover an $8\times8$ checkboard? If it is not possible enter '0' as your answer.
The units of the $L-$shaped figure are necessary to solve this problem. We have added them into the question.
Division E Problem 13: Myles, Mya and Myron entered a team competition where they each had to run $400$ meters and add up their times to get their team score. Myles was $4$ seconds faster than Myron, and Mya was $10$ seconds faster than Myron. If their total time was $181$ seconds, how many seconds did it take Myron to complete the course?
The answer response is now fixed.
Division E Problem 20: Debbie is mixing orange juice concentrate for her restaurant. The first juice concentrate is $64\%$ real orange juice. The second is only $48\%$ real orange juice. How many ounces of $48\%$ real orange juice should she use to make $1600$ ounces of $58\%$ real juice?
The answer response is now fixed.
Division M Problem 1: A goldsmith has $10$ grams of a $40\%$ gold alloy (an alloy is a mixture of metals). How many grams of pure gold should be added to make an alloy which is $60\%$ gold?
The solution was rewritten so that it is more clear and the answer is now fixed.
Division M Problem 4: There are $2$ packs of crayons available for every $5$ students at Amy's art class. How many students can share $18$ packs of crayons?
The answer response is now fixed.
Division H Problem 5: Recall that $\lfloor x\rfloor$ is the greatest integer $\leq x$. What is the sum of all solutions to $\left\lfloor x \right\rfloor^2 + \left\lfloor x \right\rfloor + 1 = 5x - 2$?
The explanation failed to account for non-integer values of $x$. Re-edited the solution and changed the answer response. Both should now be fixed.
Division H Problem 15: Suppose $f(x) = 3x+2$ and $f^{-1}(x)$ is the inverse of $f(x)$. What is $f(7) f^{-1}(-7)$?
The answer response is now fixed.
Division JV Problem 4: A shop has $8$ types of cookies and you want to buy $6$ cookies. In how many ways can you buy the cookies if you want to make sure to have an even number of each type of cookies you buy?
The solution was rewritten and the answer is now fixed.
Division JV Problem 16: Suppose $12$ people will divide and form $4$ teams of $3$ people to play two games of $3$ on $3$ basketball, a first game and a second game. If we only care about the two games being played (i.e. 'Team A vs Team B and Team C vs Team D' is the same as 'Team B vs Team A and Team D vs Team C') how many different ways can the games happen?
The solution was rewritten and the answer is now fixed.
Again, we apologize for the inaccuracies during the ZIML competition. We will keep a better eye on the questions prepared for next month's ZIML competition. Regardless, we hoped that you had fun solving these problems and are excited to have you participate again next month! Good luck to all participants and congratulations to the winners of the February 2017 ZIML Competition!
If you notice any more errors on the February 2017 ZIML Competition, please let us know. Thank you!